Estimation of standing height in spina bifida: model development and validation

Abstract

Objective

Childhood standing height has been estimated from arm span-related (height_AS) models. The authors aimed to develop and cross-validate a height_AS model in individuals with spina bifida (SB) and examine the accuracy of existing height_AS models.

Methods

Participants were individuals with sacral and low-lumbar SB (n = 14) and non-SB (n = 83), 7–16 years old. Arm span, age, sex, and group (SB vs. non-SB) were candidate height predictors. Sequential regression and leave-one-out cross-validation approaches were used for the model development (M1) and cross-validation (M1–M5). Existing models were: an SB-specific model from Polfuss et al. (M2) and non-SB specific models from Gauld et al. (M3), Mulu et al. (M4), and Zverev et al. (M5) studies.

Results

Arm span and group explained 95 % of the variance in height (R² = 0.95; p < 0.001; SEE = 3.666 cm) and were included in the M1. Mean differences between actual and estimated height were 0.0 cm (M1), 0.4 cm (M2), and 0.5 cm (M5), all not significant (p > 0.05). However, Bland-Altman analysis revealed some variability in the predictability of the models across participants with limits of agreement ranging from 7.4 to 10.9 cm. Considerable errors were observed with M3 (mean diff: −5.58 cm, 95 % CI: −1.6, −20.2 cm), and M4 (mean diff: 10.5 cm, 95 % CI: −13.8, −27.3 cm).

Conclusions

Models (M1, M2 and M5) may accurately estimate standing height in groups of children with SB. However, due to the wide limits of agreement, caution is recommended when applying these models for individual height estimations.

Introduction

Spina bifida (SB), a condition characterized by a neural tube defect, is associated with significant limitations in neuromotor and sensory systems.¹ With an estimated population prevalence of 34–81 per 100,000 live births,² it has been demonstrated that children and adolescents with SB experience several physical and cognitive conditions such as weakness or paralysis of the lower limbs,¹ lower physical fitness,³ reduced physical activity levels,⁴ sleep disorders,⁵ and impaired executive functions and attention⁶ compared with their peers without disabilities. It has further been demonstrated that this population is at higher risk for growth faltering than the general population. For example, previous studies have observed that participants with SB aged 3–14 years had lower height based on sex- and age-specific growth references.^7–9 Since linear growth is the best overall indicator of childhood well-being,¹⁰ measuring the height in children and adolescents with SB is a priority for health research and clinical practice.

Standing height is a well-recognized measure of linear growth in school-aged children and adolescents.¹¹ This measure, however, cannot be easily obtained from children and adolescents with SB who have weakness or paralysis of the lower limbs. Recumbent length is another measure for monitoring linear growth, but it can still be inappropriate due to common lower limb contractures associated with neuromuscular diseases.¹² Therefore, alternative anthropometrics for estimating the standing height, which would allow for easy application to large cohorts of children and adolescents with SB, are required.

An approach that can be easily standardized and applied within population-level linear growth monitoring involves measuring the arm span. It has been observed that arm span predicts standing height among children and adolescents without disabilities.^13–17 Past research has developed and cross-validated models for estimating standing height based on arm span (height_AS) in children and adolescents with and without disabilities.^15–18 Polfuss et al.¹⁸ developed an SB-specific standing height model that includes arm span, age, and lesion levels (sacral, lumbar, and thoracic) in a group of 418 children and adolescents with SB from the United States. In contrast, the models developed by Gauld et al.¹⁷ and Zverev et al.¹⁵ were non-SB specific and included arm span and age, while the model from Mulu et al. was non-SB specific and included only arm span. Since the models by Gauld et al.,¹⁷ Zverev et al.,¹⁵ and Mulu et al.¹⁶ were developed for children without SB, the accuracy in estimating standing height in children and adolescents with SB remains uninvestigated. Considering that children and adolescents with SB are at a higher risk for lower height compared to their peers without SB,^7–9 it is hypothesized that existing height estimation models may be biased for individuals with SB. The model by Polfuss et al.¹⁸ is anticipated to perform better in estimating standing height, as it was specifically developed for children and adolescents with SB, although it has not yet been validated for estimating linear growth in this population.

Therefore, there is a need to develop and cross-validate a height_AS model to support health professionals and researchers for population-level linear growth surveillance in this population. Additionally, it is important to compare the performance of a specific height_AS model with existing height_AS models. The aim of this study was to develop and cross-validate a height_AS model for estimating standing height in children and adolescents with SB. This study further aimed to examine the performance of an SB specific model from Polfuss et al.¹⁸ and non-SB specific models from Gauld et al.,¹⁷ Mulu et al.,¹⁶ and Zverev et al.,¹⁵ for estimating standing height in children and adolescents with SB.

Methods

Participants

The authors recruited participants with and without SB at the Rehabilitation Center at the University of São Paulo, Ribeirão Preto, Brazil, and the surrounding communities. Inclusion criteria for this study were: 1) participants with and without SB with ages following the school-aged World Health Organization definition (i.e., 5–19 years old); 2) participants who were ambulatory and did not have any orthopedic conditions that could affect the measurement of height and horizontally outstretched arms span; and 3) ability to understand procedures. The protocol of this study was approved by the ethics committee of the Ribeirão Preto Medical School, University of São Paulo, with the Declaration of Helsinki followed during all study procedures. The authors obtained written informed consent from all participants and their parents/guardians. The authors included a total of 83 participants without SB (46 males and 37 females; ages 12.13 ± 2.75 years) and 14 participants with SB (5 males and 9 females; ages 10.51 ± 2.23 years). All participants with SB had spinal cord injury at the sacral (n = 11) and low lumbar (n = 3) levels.

Anthropometric measures

Standing height and arm span were obtained from all participants by experienced technicians following standardized procedures. Standing height was measured with a stadiometer to the nearest 0.1 cm. Arm span was measured as the distance between the tips of the middle fingers with arms outstretched horizontally using a metal tape to the nearest 0.1 cm, with participants in a seated position.

Data analyses

Data analyses were performed in SPSS Statistics 22 (IBM, Armonk, NY). The alpha level was set at 0.05. The normality of data was examined using the Shapiro–Wilk test, histograms, boxplots, and Q-Q-plots. Comparisons of demographic and anthropometric variables between participants with and without SB were examined with an independent-sample t-test and Mann-Whitney U test. Spearman rho rank-order correlation (r_s) was used to examine the bivariate association between height and arm span. The height prediction model was developed using sequential multiple regression. Independent variables were initially entered in the regression following this step sequence: 1) arm span; 2) age; 3) sex; and 4) group (SB vs. non-SB). The coefficient of determination (R²) and standard error of estimate (SEE) were used to examine the goodness-of-fit of the final model. The present height estimation model was cross-validated using the leave-one-out cross-validation approach. Specifically, this approach involved splitting the whole sample (n = 97) into training samples (i.e., all participants except one; n = 96) and validation samples (i.e., the omitted participant; n = 1). This process was made in two steps: in step 1, the authors ran a model (model 1) using a first training sample (sample 1a; participant ID 2, 3…97); and in step 2, the authors used the resulting regression coefficients from the model 1 to estimate the height in the first validation sample (sample 1b; participant ID 1). The authors repeated steps 1–2 involving the resting modeling process for all possible subsamples of 97 participants. Agreement between actual and estimated height was examined with mean absolute error (MAE), root mean square error (RMSE), and Bland-Altman Analysis. Additionally, the Pearson correlation coefficient (r) was used to examine the associations between actual and estimated height. The square of this Pearson correlation coefficient was compared to the R² of the original regression model for the evaluation of the generalizability of the regression model as previously recommended.¹⁹ Moreover, the authors compared the actual and estimated mean height with paired samples t-test.

Additionally, the authors estimated the height of the present participants with SB using an SB specific model from Polfuss et al.¹⁸ (M2_{Polfuss et al.}) and three non-SB specific models from Gauld et al.¹⁷ (M3_{Gauld et al.}), Mulu et al.¹⁶ (M4_{Mulu et al.}), and Zverev et al.¹⁵ (M5_{Zverev et al.}):

M2_{Polfuss et al.}¹⁸

M3_{Gauld et al.}¹⁷

M4_{Mulu et al.}¹⁶

M5_{Zverev et al.}¹⁵

For all models, arm span is in cm and age is in years.

The performance of the height estimated by these models was evaluated with MAE, RMSE, and Bland–Altman analysis.

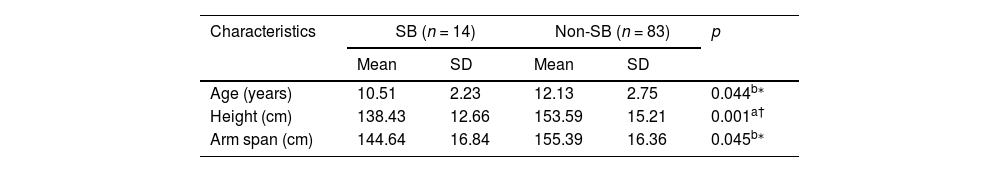
Results

Table 1 presents the age and anthropometric characteristics of the participants with and without SB. There were significantly lower age, height, and arm span in participants with SB than in non-SB (p ≤ 0.05; Table 1).

Table 1.

Age and anthropometric characteristics of children and adolescents with and without spina bifida.

Characteristics	SB (n = 14)		Non-SB (n = 83)		p
	Mean	SD	Mean	SD
Age (years)	10.51	2.23	12.13	2.75	0.044^b^⁎
Height (cm)	138.43	12.66	153.59	15.21	0.001^a^†
Arm span (cm)	144.64	16.84	155.39	16.36	0.045^b^⁎

Note: data are presented as mean and standard deviation (SD), and frequencies (n); SB, spina bifida; non-SB, without spina bifida.

a

Independent t-test.

b

Mann-Whitney U test.

⁎

p <0.05.

†

p < 0.01.

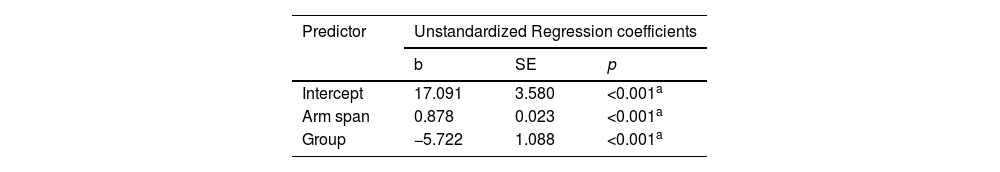
Significant bivariate correlation was observed between actual height and arm span in overall (r_s = 0.96; p < 0.001), non-SB (r_s = 0.97; p < 0.001), and SB (r_s = 0.93; p < 0.001) groups. Sequential regression indicated that arm span was a significant predictor of height (R² = 0.93; p < 0.001; SEE = 4.150 cm). There were no additional contributions of age (ΔR² = 0.002; p = 0.083) and sex (ΔR² = 0.000; p = 0.459) to the model. Group (SB vs. non-SB) had an additional contribution to the height (ΔR² = 0.016; p < 0.001). Therefore, the final model (M1_Present) included arm span and group (R² = 0.95; p < 0.001; SEE = 3.666 cm). Unstandardized regression coefficients are presented in Table 2. The M1_Present for the estimation of standing height in participants with and without SB was:

Table 2.

Regression model for the estimation of height based on arm span and group (SB vs. non-SB).

Predictor	Unstandardized Regression coefficients
	b	SE	p
Intercept	17.091	3.580	<0.001^a
Arm span	0.878	0.023	<0.001^a
Group	−5.722	1.088	<0.001^a

Note: b, unstandardized coefficient; SE, standard error.

a

p < 0.001.

M1_Present:

For this model, the arm span is in cm, and the group is 0 = non-SB and 1 = SB.

The M1_Present was cross-validated based on strong association and non-significant differences between actual height and estimated height_AS, as well as small MAE, RMSE, and differences in Bland–Altman analysis (Table 3 and Figure 1). Specifically, the authors observed a strong significant association between actual and height_AS estimates in the SB group (Table 3 = 0.95; p < 0.001). The high generalizability of the model was indicated by small differences between the square of this Pearson correlation coefficient (0.90) with the coefficient of determination of the model (0.95) (Table 3). Differences between actual height and estimated height_AS in the SB group were not significant (actual height: 138.43 ± 12.66 cm; and estimated height_AS: 138.44 ± 15.05 cm; p = 0.993) (Table 3). MAE was 4.32 ± 2.20 cm and RMSE was 1.83 cm (Table 3). In the Bland–Altman plot, the mean difference between actual height and estimated height_AS was close to zero (mean error: −0.01 cm; 95 % CI: 9.76, −9.81 cm; Figure 1, and Table 3).

Table 3.

Performance of arm span-related models for the estimation of height among participants with and without SB of the present study.

Group	Model	Mean (SD)^a	MAE^a	RMSE^a	Mean diff (95 % CI)^a^,^b	r	r^2
Non-SB	Actual	153.59 (15.21)	–	–	–	–	–
Non-SB	M1Present	153.60 (14.37)	2.92	3.28	−0.01 (7.00, −7.00)	0.97^d	0.94
SB	Actual	138.43 (12.66)	–	–	–	–	–
SB	M1Present	138.44 (15.05)	4.32	1.83	−0.01 (9.76, −9.81)	0.95^d	0.90
SB	M2Polfuss et al.	138.03 (14.77)	4.41	1.89	0.40 (10.87, −9.29)	0.94^d	0.88
SB	M3Gauld et al.	144.01 (13.84)^c	5.72	2.74	−5.58 (−1.63, −20.25)	0.94^d	0.88
SB	M4Mulu et al.	148.90 (10.93)^c	10.47	4.17	−10.47 (−13.77, −27.28)	0.97^d	0.94
SB	M5Zverev et al.	138.91 (14.14)	3.58	1.57	−0.48 (7.42, −9.31)	0.96^d	0.92

Note: SB, participants with spina bifida; Non-SB, participants without spina bifida; MAE, mean absolute error; RMSE, root mean square error.

a

values are in cm.

b

mean difference and 95 % confidence intervals from Bland–Altman analysis.

c

significance (p < 0.01) between estimated and actual height in paired t-tests.

d

significance (p < 0.001) for Pearson correlations between actual and estimated height; M1_Present - the model developed in this study, M2_{Polfuss et al.} - the model from Polfuss et al.,¹⁸ M3_{Gauld et al.} - the model from Gauld et al.,¹⁷ M4_{Mulu et al.} - the model from Mulu et al.,¹⁶ and M5_{Zverev et al.} - the model from Zverev et al.¹⁵

Figure 1.

SB, participants with spina bifida; Non-SB, participants without spina bifida; Bland–Altman plot of the difference between actual and estimated height (y-axis) against their average (x-axis) using the M1_Present - the model developed in this study, M2_{Polfuss et al.} - the model from Polfuss et al.,¹⁸ M3_{Gauld et al.} - the model from Gauld et al.,¹⁷ M4_{Mulu et al.} - the model from Mulu et al.,¹⁶ and M5_{Zverev et al.} - the model from Zverev et al.¹⁵ among participants with SB (cross marker) and without spina bifida (grey circle marker); dashed lines show 95 % limits of agreement; solid line represents the mean difference.

Predictive performance results for previously published height_AS models are presented in Table 3 and Figure 1. The authors observed that the M2_{Polfuss et al.} and M5_{Zverev et al.} models had better predictive performance for the estimation of standing height compared to M3_{Gauld et al.} and M4_{Mulu et al.} models. Specifically, the authors observed that differences between actual and estimated height were significant using M3_{Gauld et al.} (mean diff: −5.58 cm, p < 0.01) and M4_{Mulu et al.} (mean diff: −10.47 cm, p < 0.01) models and non-significant using M2_{Polfuss et al.} (mean diff: −0.40 cm, p = 0.775) and M5_{Zverev et al.} (mean diff: −0.48 cm, p = 0.679) models (Table 3). Moreover, M5_{Zverev et al.} model had narrower limits of agreement (M5_{Zverev et al.} 95 % CI: 7.42, −9.31 cm) compared to other models in Bland-Altman analysis (Table 3 and Figure 1).

Discussion

This study developed and cross-validated a height_AS model for the estimation of standing height among children and adolescents with SB. This study further observed that M2_{Polfuss et al.} (SB-specific model) and M5_{Zverev et al.} (non-SB specific model) had the similar predictive ability to estimate standing height. Developed and validated specific prediction models would be useful for researchers in estimating standing height among children and adolescents with SB.

The present study demonstrated that arm span had a strong bivariate association with height among participants with SB (r = 0.93) and without SB (r = 0.97). The present observations are consistent with previous data that indicated that arm span was moderate and strongly correlated with height (r = 0.71 to 0.98) among children and adolescents aged 7–18 years without disabilities.^13–16 Moreover, this study observed that arm span alone explained 93 % of the variance in height in the present sample. A finding that should be considered, however, is that arm span alone did not explain 7 % of the variance in standing height in this study. There is evidence that age and sex are additional significant predictors of height among children and adolescents from the general population. However, the authors found no contributions of these variables to this study's model, necessitating an examination of other potential predictors. In the present study, the authors observed that the group (SB vs. non-SB) explained an additional 2 % of the variance in standing height. The effect of the group was expected given that children and adolescents with SB are at higher risk for short height.^7–9 Taken together, arm span and group were significant predictors of height and were therefore included in the final model for the estimation of standing height. The next step involved cross-validating this arm span-related model.

The authors provided evidence that the M1_Present was valid for estimating standing height in the present participants with and without SB. Errors based on MAE and RMSE were relatively small in the whole cross-validation process. Moreover, there was a high generalizability of the model as indicated by minimal differences in the associations between training and validation samples. Additionally, the authors observed non-significant differences between actual and estimated height using this model. These findings agree with a previous cross-validation study that indicated non-significant mean differences and strong associations between actual height and estimated height_AS among Malawians aged 6–15 years (the M5_{Zverev et al.} model).¹⁵ Another important finding was that the model produced a zero mean difference and somewhat wide 95 % limits of agreement in Bland-Altman analysis.

Another important finding in this study was that the M1_Present model had a similar predictive ability in comparison with an SB specific (M2_{Polfuss et al.}) and a non-SB specific (M5_{Zverev et al.}) model for standing height estimation of children and adolescents with SB. A closer inspection of the M1_Present and M2_{Polfuss et al.} Bland-Altman plots, however, indicate a trend from overestimation at lower average heights to underestimation at higher average heights. Moreover, the authors observed that M5_{Zverev et al.} model had a minimal trend and smaller MAE, RMSE, and 95 % limits of agreement in Bland–Altman analysis compared to M1_Present and M2_{Polfuss et al.} models. A consideration is that the M5_{Zverev et al.} model was derived from a sample with somewhat small differences in anthropometrics when compared to this sample with SB. For example, the authors found that the present sample had mean arm span and height z-scores varying from −0.6 to −1.5 based on the data from the Zverev study. It was further observed considerable mean height differences between actual, M3_{Gauld et al.,} and M4_{Mulu et al.} models, with limits of agreement reaching −27 cm. A possible explanation for large differences between M3_{Gauld et al.,} and M4_{Mulu et al.} models may include variations in age, height, and arm span between samples. It is additionally important to note that M3_{Gauld et al.} and M5_{Zverev et al.} models included age in the sex-specific models. By comparison, the authors observed that age had no contributions to the present regression model after accounting for the effect of arm span. Yet, the development of sex-specific models was not possible in this study because the sample size was not adequate to capture the influence of the group – future research is needed to address this issue. Another important consideration is that existing height_AS models evaluated in this study were developed in children and adolescents from the United States, Ethiopia, Africa, and Australia. Research has demonstrated that linear growth, especially height, varies considerably in children and adolescents from different geographical regions.²⁰

Measuring standing height from the height_AS has implications for research and clinical practice. Taken together, M1_Present, M2_{Polfuss et al.}, and M5_{Zverev et al.} models may be used with confidence in research contexts, as these models demonstrated minimal errors on a group basis, but caution regarding sample profiles and measurement protocols is required. Applying these models would support researchers in evaluating linear growth in participants with SB with posture difficulties due to weakness or paralysis of the lower limbs. The length of body segments has been widely used for measuring height among individuals with physical disabilities.²¹ Additionally, the present model may be useful for supporting large-scale growth surveillance research among participants with SB. Past research has demonstrated that children and adolescents with SB may be at high risk for short height.^7–9 It is recognized that height is a fundamental predictor of overall health in children and adolescents.¹⁰ However, due to the wide limits of agreement, caution is advised when using M1_Present, M2_{Polfuss et al.}, and M5_{Zverev et al.} models for the evaluation of linear growth in clinical practice, as these models may not demonstrate adequate predictive performance on an individual basis. Moreover, future research with large sample sizes is necessary to test the ability of the presented height_AS models to identify individuals with SB presenting with below- and above-average standing height based on the interpolation using sex- and age-specific values from growth references such as those from Center from Centers for Disease Control and Prevention and the World Health Organization. Additionally, M3_{Gauld et al.,} and M4_{Mulu et al.} models are not recommended for clinical and research purposes, as the differences observed were beyond the acceptable limits of agreement.

This study has some limitations and strengths that should be considered. Limitations include cross-sectional data from a small sample of participants with SB. Additionally, there were no participants with spinal cord injury at mid- and high-lumbar, and thoracic levels. This study also focused on a narrow age range of individuals with spina bifida (7–16 years old), limiting the applicability of the findings to those outside this age group. Finally, although this model was validated based on leave-one-out cross-validation approach, future research should consider cross-validating the model in other samples. Strengths of this study include measuring height in a standing position. This would allow comparison of standing height data of children with SB with growth standards following standardized procedures. Moreover, the authors cross-validated the present model including two simple variables and examined the performance of existing SB and non-SB specific models, enabling the practical application of the models in epidemiological research contexts.

This study indicated that arm span was strongly associated with standing height in children and adolescents with SB. A model including arm span and group was developed and cross-validated for estimating standing height in individuals with sacral and low-lumbar SB aged 7–16 years. Existing models (M2_{Polfuss et al.}, and M5_{Zverev et al.}) had similar height predictability compared to the present model (M1_Present). These models may be useful to researchers for linear growth surveillance in children and adolescents with SB. However, due to the wide limits of agreement, the authors recommend caution if applying these models for individual estimation of standing height.

Funding

This study was supported by “São Paulo Research Foundation (FAPESP)” [grant numbers: 2017/17596-4; 2022/00099-6]; “Coordenação de Aperfeiçoamento de Pessoal de Nível Superior” (Capes) [scholarship numbers: 88887.586702/2020-00; 001] and “Fundação de Apoio ao Ensino, Pesquisa e Assistência do Hospital das Clínicas da Faculdade de Medicina de Ribeirão Preto da Universidade de São Paulo” (FAEPA).

Acknowledgments

The authors wish to thank the volunteers who participated in this study.